Welcome to the resource topic for 2022/226
Title:
To Label, or Not To Label (in Generic Groups)
Authors: Mark Zhandry
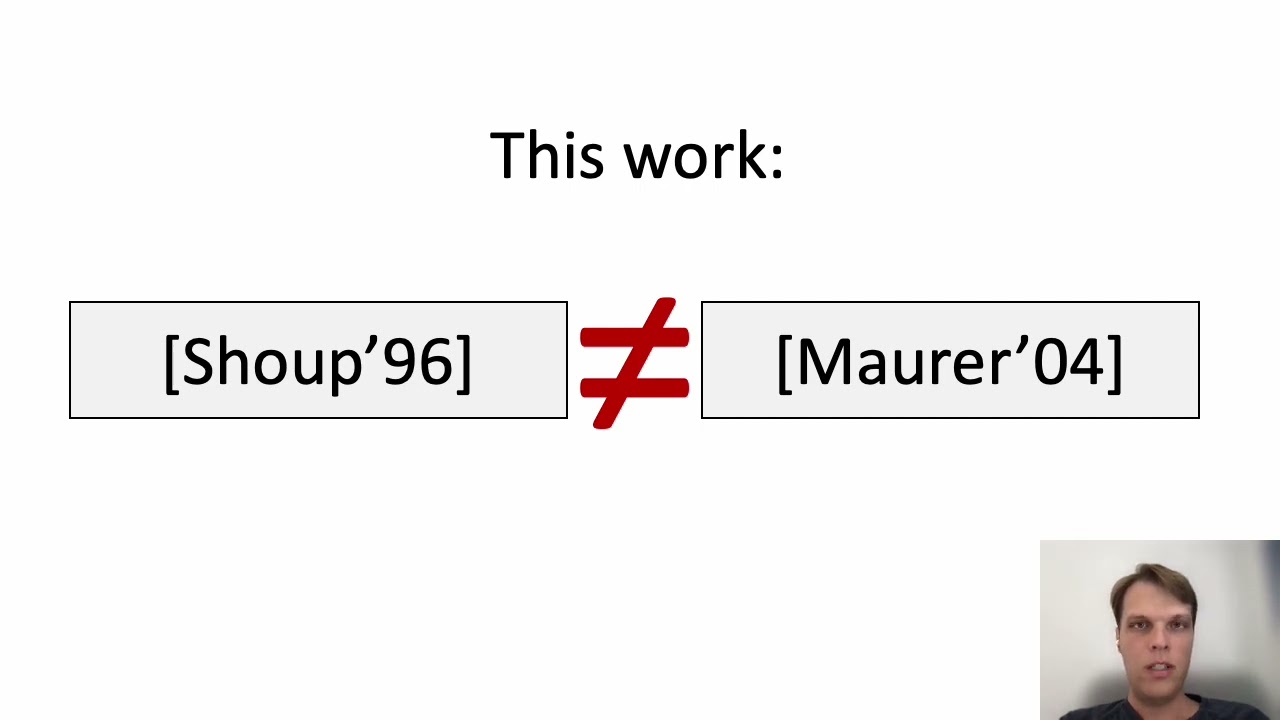
Abstract:Generic groups are an important tool for analyzing the feasibility and in-feasibility of group-based cryptosystems. There are two distinct wide-spread versions of generic groups, Shoup’s and Maurer’s, the main difference being whether or not group elements are given explicit labels. The two models are often treated as equivalent. In this work, however, we demonstrate that the models are in fact quite different, and care is needed when stating generic group results: - We show that numerous textbook constructions are not captured by Maurer, but are captured by Shoup. In the other direction, any construction captured by Maurer is captured by Shoup. - For constructions that exist in both models, we show that security is equivalent for “single stage” games, but Shoup security is strictly stronger than Maurer security for some “multi-stage” games. - The existing generic group un-instantiability results do not apply to Maurer. We fill this gap with a new un-instantiability result. - We explain how the known black box separations between generic groups and identity-based encryption do not fully apply to Shoup, and resolve this by providing such a separation. - We give a new un-instantiability result for the algebraic group model.
ePrint: https://eprint.iacr.org/2022/226
Talk: https://www.youtube.com/watch?v=sLszAQ47YW8
Slides: https://iacr.org/submit/files/slides/2022/crypto/crypto2022/3/slides.pptx
See all topics related to this paper.
Feel free to post resources that are related to this paper below.
Example resources include: implementations, explanation materials, talks, slides, links to previous discussions on other websites.
For more information, see the rules for Resource Topics .