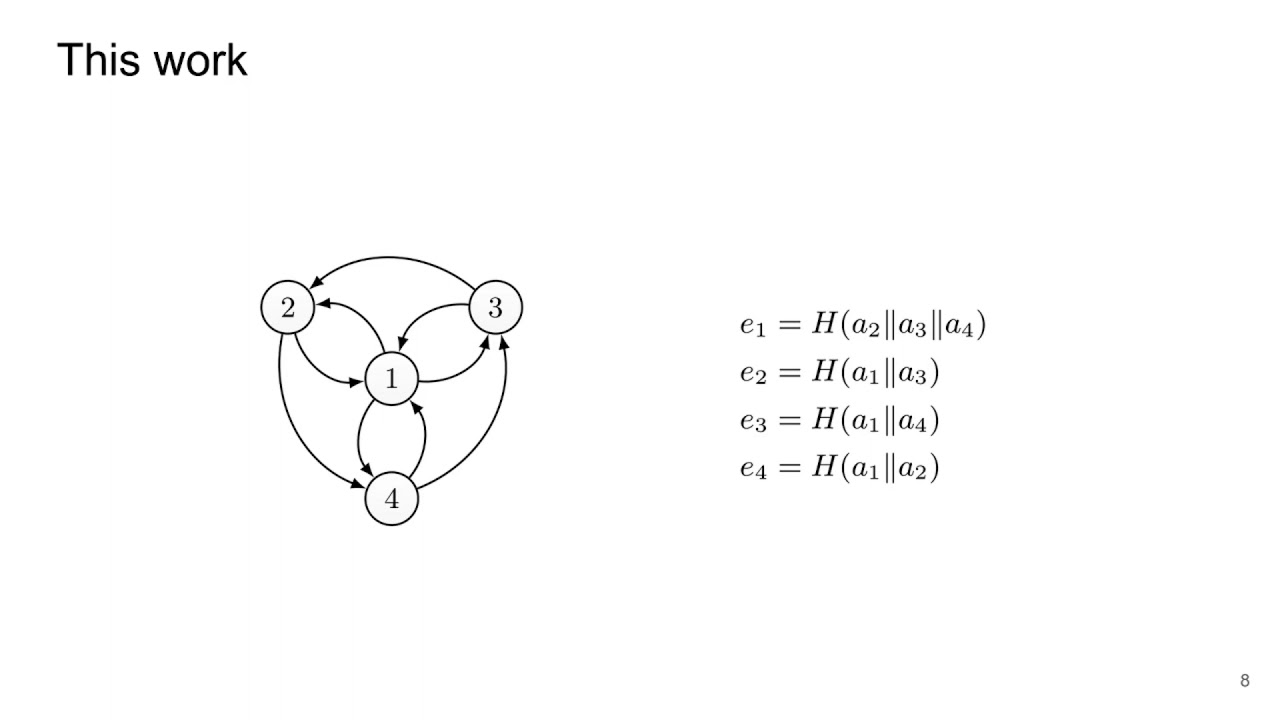Welcome to the resource topic for 2021/135
Title:
Acyclicity Programming for Sigma-Protocols
Authors: Masayuki Abe, Miguel Ambrona, Andrej Bogdanov, Miyako Ohkubo, Alon Rosen
Abstract:Cramer, Damgård, and Schoenmakers (CDS) built a proof system to demonstrate the possession of subsets of witnesses for a given collection of statements that belong to a prescribed access structure P by composing so-called sigma-protocols for each atomic statement. Their verifier complexity is linear in the size of the monotone span program representation of P. We propose an alternative method for combining sigma-protocols into a single non-interactive system for a compound statement in the random oracle model. In contrast to CDS, our verifier complexity is linear in the size of the acyclicity program representation of P, a complete model of monotone computation introduced in this work. We show that the acyclicity program size of a predicate is never larger than its de Morgan formula size and it is polynomially incomparable to its monotone span program size. We additionally present an extension of our proof system, with verifier complexity linear in the monotone circuit size of P, in the common reference string model. Finally, considering the types of statement that naturally reduce to acyclicity programming, we discuss several applications of our new methods to protecting privacy in cryptocurrency and social networks.
ePrint: https://eprint.iacr.org/2021/135
Talk: https://www.youtube.com/watch?v=XTDPh7iVu-A
See all topics related to this paper.
Feel free to post resources that are related to this paper below.
Example resources include: implementations, explanation materials, talks, slides, links to previous discussions on other websites.
For more information, see the rules for Resource Topics .