Welcome to the resource topic for 2021/1228
Title:
Computational Robust (Fuzzy) Extractors for CRS-dependent Sources with Minimal Min-entropy
Authors: Hanwen Feng, Qiang Tang
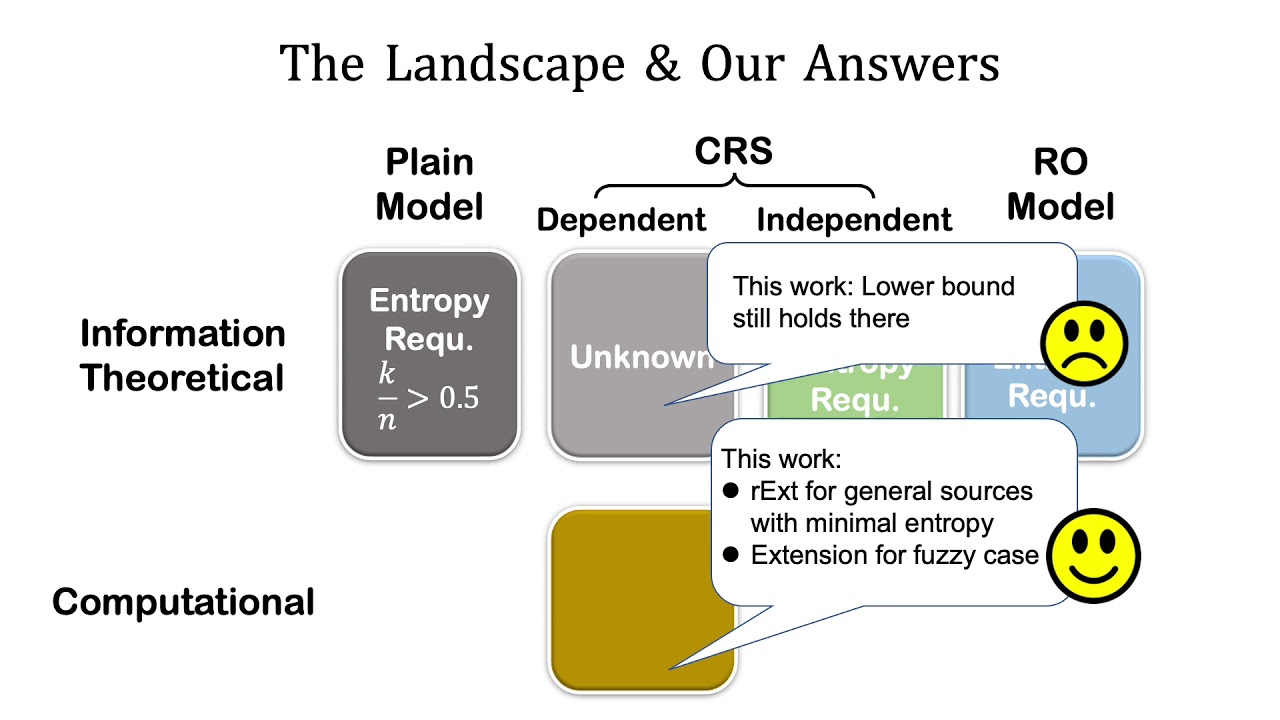
Abstract:Robust (fuzzy) extractors are very useful for, e.g., authenticated key exchange from a shared weak secret and remote biometric authentication against active adversaries. They enable two parties to extract the same uniform randomness with a helper'' string. More importantly, they have an authentication mechanism built in that tampering of the helper’’ string will be detected. Unfortunately, as shown by Dodis and Wichs, in the information-theoretic setting, a robust extractor for an (n,k)-source requires k>n/2, which is in sharp contrast with randomness extractors which only require k=\omega(\log n). Existing works either rely on random oracles or introduce CRS and work only for CRS-independent sources (even in the computational setting). In this work, we give a systematic study about robust (fuzzy) extractors for general CRS {\em dependent} sources. We show in the information-theoretic setting, the same entropy lower bound holds even in the CRS model; we then show we {\em can} have robust extractors in the computational setting for general CRS-dependent source that is only with minimal entropy. We further extend our construction to robust fuzzy extractors. Along the way, we propose a new primitive called \kappa-MAC, which is unforgeable with a weak key and hides all partial information about the key (both against auxiliary input); it may be of independent interests.
ePrint: https://eprint.iacr.org/2021/1228
Talk: https://www.youtube.com/watch?v=LR8y6HRZrK4
See all topics related to this paper.
Feel free to post resources that are related to this paper below.
Example resources include: implementations, explanation materials, talks, slides, links to previous discussions on other websites.
For more information, see the rules for Resource Topics .