Welcome to the resource topic for 2020/282
Title:
The Measure-and-Reprogram Technique 2.0: Multi-Round Fiat-Shamir and More
Authors: Jelle Don, Serge Fehr, Christian Majenz
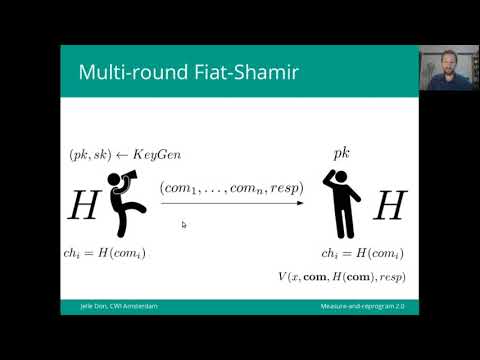
Abstract:We revisit recent works by Don, Fehr, Majenz and Schaffner and by Liu and Zhandry on the security of the Fiat-Shamir transformation of \Sigma-protocols in the quantum random oracle model (QROM). Two natural questions that arise in this context are: (1) whether the results extend to the Fiat-Shamir transformation of multi-round interactive proofs, and (2) whether Don et al.'s O(q^2) loss in security is optimal. Firstly, we answer question (1) in the affirmative. As a byproduct of solving a technical difficulty in proving this result, we slightly improve the result of Don et al., equipping it with a cleaner bound and an even simpler proof. We apply our result to digital signature schemes showing that it can be used to prove strong security for schemes like MQDSS in the QROM. As another application we prove QROM-security of a non-interactive OR proof by Liu, Wei and Wong. As for question (2), we show via a Grover-search based attack that Don et al.'s quadratic security loss for the Fiat-Shamir transformation of \Sigma-protocols is optimal up to a small constant factor. This extends to our new multi-round result, proving it tight up to a factor that depends on the number of rounds only, i.e. is constant for any constant-round interactive proof.
ePrint: https://eprint.iacr.org/2020/282
Talk: https://www.youtube.com/watch?v=HhiVcFpJE38
See all topics related to this paper.
Feel free to post resources that are related to this paper below.
Example resources include: implementations, explanation materials, talks, slides, links to previous discussions on other websites.
For more information, see the rules for Resource Topics .